“Why can’t I use the model method to solve this problem? I can still get the answer!” This is a common complaint received by secondary school Math teachers. Some have even resorted to banning the use of the model method in class because they believe it prevents students from learning to use letter-symbolic algebra. But is this belief valid?

To solve word problems, it is important for students to represent the information presented in the word problems. Students as young as Primary 2 are taught to construct model drawings to help them visualize the word problems.

Because of the familiarity with the model method, many students continue to use it even at the secondary school level, where they are taught to use the more abstract letter-symbolic algebra.¹ (Ng, 2003)

However, many secondary school teachers are concerned that continued use of the model method may prevent students from learning letter-symbolic algebra, which is the preferred tool.

Letter-symbolic algebra is also the language of higher mathematics, making it the gateway for students who want to further their mathematical study. Letter-symbolic algebra can be used to solve all types of algebra word problems, while the model method is limited in its applications.

When efforts to discourage the use of the model method fail, some teachers resort to desperate measures such as banning the use of the model method in their classrooms.

But does the model method really hinder students from learning symbolic algebra?

Model Method versus Letter-symbolic Algebra

The model method and letter-symbolic algebra are methods that can be used to solve algebraic word problems. The main differences are in the manner in which the unknowns are represented and how the value of the unknown is evaluated.²

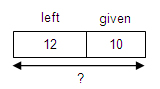
The following, taken from a Secondary 1 Math textbook, is an example of a question that can solved using both the model method and symbolic algebra.

Using the Model Method as a Bridge

When the model method was introduced to the curriculum, it was not intended as a tool to help students learn symbolic algebra. However, continuous use of the model method by lower secondary school students demands that teachers take another look at how students’ knowledge of the model method can be used to help students learn letter- symbolic algebra.

In a study by Associate Professor Ng Swee Fong, teachers found that the model method and letter-symbolic algebra are related (Ng, 2003). A word problem captured in a model diagram may be converted to algebraic equations and vice versa.

For students to make the transition to letter-symbolic algebra, teachers can help them by asking a series of appropriately targeted questions such as:

• In the model method what do rectangles represent?
• Instead of rectangles, can other objects be used to take over the role of the rectangles?
• Can letters of the alphabet be used instead?

So, instead of banning the use of the model method, secondary school teachers can activate students’ prior knowledge of the model method and use it as a bridge to teach letter-symbolic algebra.

What Can Be Done?

Although the connection between the two models was taught to all pre-service teachers, the significance of this knowledge may not be appreciated at that point as pre-service teachers lack actual classroom experience.

Dr Ng and her colleagues (2006) suggest that it may be more useful to target this knowledge at lower secondary school math teachers, who are currently facing the model method-symbolic algebra dilemma.

The important thing is not to stop students from using any method to solve a problem. With understanding comes appreciation. Once students understand what they are learning, they can better appreciate the usefulness and importance of letter-symbolic algebra. Then, they can choose the most effective method to solve problems.

Notes

1. In the current curriculum, students learn the model method first in primary school, before being introduced to abstract letter-symbolic algebra in secondary school.
2. In the model method, rectangles are used to represent unknowns and the arithmetic method is used to evaluate the unknown. In letter-symbolic algebra, letters represent unknowns and the value of the unknown is evaluated using transformational procedures, which maintain the equivalence of an equation.
3. Using letter-symbolic algebra, the answer to this question, expressed as an algebraic equation, looks like this: x  – 10 + 10 = 12 + 10 = 22

References
Ng, S. F. (2003). How secondary two express stream students used algebra and the model method to solve problems. The Mathematics Educator, 7(1), 1-17.

Ng, S. F., Lee, K., Ang, S. Y., & Khng, F. (2006). Model method: Obstacle or bridge to learning symbolic algebra. In W. D. Bokhorst-Heng, M. D. Osborne, & K. Lee (Eds.), Redesigning pedagogies: Reflections on theory and praxis (pp. 227-242). Rotterdam, Netherlands: Sense Publishers.
Sin, K. M., Chip, W. L, & Ng, S. B. (2006). Mathematics matters. Singapore: EPB Pan Pacific.

Further reading
Ng, S. F. (2009, June). What is algebraic about the model method? Paper presented at the international conference on Redesigning Pedagogy: Designing New Learning Contexts for a Globalising World, National Institute of Education, Nanyang Technological University, Singapore.

Read more about the Teaching and Learning Mathematical Word Problems: A Comparison of the Model and Symbolic Method project by the Centre for Research in Pedagogy and Practice (CRPP). The project is led by Associate Professors Kerry Lee and Ng Swee Fong, from CRPP and the Mathematics and Mathematics Education Academic Group, respectively.