by Chan Chun Ming Eric

Problem-based learning as an instructional approach helps pupils to develop mathematical thinking skills. This article shows us how it can be used to teach mathematical modelling by using a real-life task.

In a problem-based learning (PBL) session, pupils work in small collaborative groups to solve a task situated in the real world, in this case a modelling task. The teacher functions as a cognitive coach while pupils develop, test and refine their models towards goal resolution.

Understanding the Use of PBL

In this article, mathematical modelling takes on a models-and-modelling perspective (Lesh & Doerr, 2003), which asserts that pupils develop conceptual representations or models that are expressed using spoken language, written symbols, concrete materials, diagrams, pictures, or other representational media.

When pupils are given non-trivial problems to solve – ones that relate to their lives – they develop models that are continually being projected onto the external world. These models are given mathematical meanings as the pupils interpret and refine them to solve the problem.

For example, when pupils identify the quantities and variables in a problem and establish relationships between them, they are using mathematical knowledge to give meaning to the problem. In doing so, they are developing a mathematical model and using it as a tool for thinking.

Expressions of such modelling include pupils’ ability to aggregate scores; to weigh or rank data for decision making; or to generate tables, graphs or lists for comparing, combining or eliminating data.

PBL in the Math Classroom

The use of a PBL platform to drive the learning of mathematical modelling is a fitting instructional approach (Hjalmarson & Diefes-Dux, 2008). Because the design of the modelling tasks is guided by modelling principles (Lesh, Cramer, Doerr, Post, & Zawojewski, 2003), the type of tasks used in a problem-based setting reflects reality.

PBL tasks thus require pupils to construct knowledge, self-assess, make their thinking visible, adapt or transfer ideas to other similar situations, and establish relationships between variables in a situation.

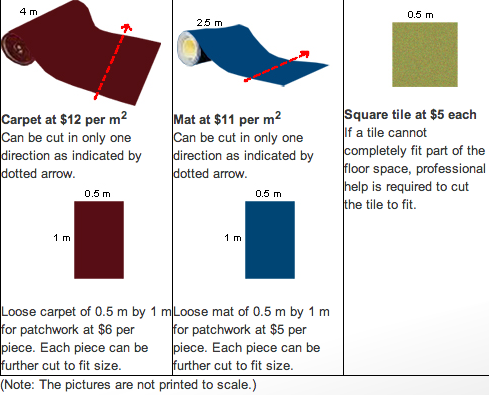
An example of a PBL task is the floor-modelling problem. It is a modification and expansion of a similar modelling task by Gravemeijer, Pligge and Clarke (1998). This task is particularly suitable for upper primary pupils.

The Problem-solving Process

Understanding the goal of a task

In this problem, pupils have to determine an appropriate choice of floor-covering material and its layout design for covering the floor of a study room.

The goal of the task lies in the statement: “Explain clearly and mathematically your best choice and how you arrive at your decision.” In solving the problem, pupils need to unpack the meaning of “best choice”.

There are two main expectations related to the pupils’ development of models:

1. The construction of layout designs, which requires a manipulation of the dimensions of the floor-covering materials to fit the dimensions of the study room; and
2. The ability to associate area and cost relationships.

The problem also presents opportunities for pupils to factor in personal knowledge or experiences and make assumptions.

Considering different ways of solving the task

A plausible solution model would be one where the pupils are able to attain a layout design that is value for money and that would fit the floor dimensions. Pupils can be encouraged to determine if they could optimize their solutions through cost and material savings.

As there are multiple ways to solve the problem, teachers need to acquaint themselves with the task first. This will help them to anticipate the various possible layout designs (models) and orientations of floor coverings.

This preparation will enable the teachers not only to make better sense of the pupils’ mathematical reasoning when solving the problem, but also to help them facilitate the session more assuredly.

Outcomes for learning

This modelling task was one of several tasks used to investigate Primary 6 pupils’ mathematical modelling process in a PBL setting.

It was found that the pupils were able to develop various layout designs as they worked on the dimensions and orientations of the materials towards covering the floor. In doing so, they engaged in manipulating geometrical and measurement aspects.

The pupils were able to establish area-cost relationships and demonstrated their ability to relate cost aspects (cost per unit area).

The pupils also acquired new learning through engaging in collaborative discourse. As they worked to refine their models, some pupils were able to improve on their initial models, resulting in further cost and material savings.

A Promising Method

When pupils are engaged in mathematical modelling tasks in a PBL setting, the interaction between pupils and the teacher produces a learning situation where cognitive immersion takes place.

PBL is in stark contrast to solving “tidy” problems found in textbooks, where there are assured ways of finding the solution, involving neat numerical figures. In a PBL setting, pupils develop problem-solving skills and habits of mind that are valued in the mathematics curriculum.

Because of the nature of the task, which requires pupils to test and revise their designs to refine their models, a high demand is placed on their metacognitive capabilities. Situating mathematical modelling in a PBL setting therefore holds promise as an excellent platform for developing pupils’ mathematical thinking.

References
Gravemeijer, K., Pligge, M. A., & Clarke, B. (1998). Reallotment. In National Center for Research in Mathematical Science Education & Freudenthal Institute (Eds.), Mathematics in context. Chicago: Encyclopaedia Britannica.

Hjalmarson, M., & Diefes-Dux, H. (2008). Teacher as designer: A framework for teacher analysis of mathematical model-eliciting activities. Interdisciplinary Journal of Problem-based Learning, 2(1), 58-78.

Lesh, R., Cramer, K., Doerr, H. M., Post, T., & Zawojewski, J. S. (2003). Model development sequences. In R. Lesh & H. M. Doerr (Eds.), Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching (pp. 35-58). Mahwah, NJ: Lawrence Erlbaum Associates.

Lesh, R., & Doerr, H. M. (2003). Foundations of a models and modeling perspective on mathematics teaching, learning, and problem solving. In R. Lesh & H. M. Doerr (Eds.), Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching (pp. 3-34). Mahwah, NJ: Lawrence Erlbaum Associates.