Are You Game Enough?

by Joseph B. W. Yeo

Children love games! But it can be more than just fun and games when mathematically rich games are used in the classroom. Learn how games can help your students acquire the skills of mathematical investigation.

Article highlights

How can mathematically rich games aid in learning?
Do students know what and how to investigate when faced with a math problem?
What are the thinking processes students use in investigating?

Many math educators believe in making math real to students. Playing mathematically rich games is one way to engage both their hearts and minds.

Such games are very real to students because the outcome – whether they win or lose – matters to them (Ainley, 1988). They may become more interested in looking for a way to win the game (Civil, 2002).

A Winning Strategy

The fact is, we all want to win! And when people don’t know how to solve a problem, they will start investigating and exploring various solutions. Problem solving and investigation are essential skills in our daily lives (Carraher & Schliemann, 2002).

Likewise, finding a winning strategy for a game involves the application of problem-solving heuristics. For example, we can solve problems by working backwards or by considering all possible scenarios.

I distinguish between a sure-win strategy, which will ensure a win for a player, and a winning strategy that maximizes the chance of winning for a player if a sure-win strategy does not exist, such as in the game Fifteen (described below).

Investigating Mathematical Investigation

In a recent study, I used mathematical games to examine the nature and development of cognitive and metacognitive processes when students engage in mathematical investigation.

A group of 20 Secondary 2 students was presented with a game called Fifteen (Mason, Burton, & Stacey, 1985). The rules of the game are simple:

Place 9 discs marked with the digits 1 to 9 on the table.
Two players take turns to pick one disc from the table.
The first player to obtain the sum of 15 among any 3 of his discs wins.

The students were tasked to explore and investigate. What they were not told is that there is a winning strategy.

I wanted to see if they knew what and how to investigate, and if they understood what a winning strategy or a sure-win strategy is, among other things.

Knowing How to Start

When faced with this game, most of the students did not even know where to start. The idea of finding a winning or sure-win strategy was alien to most of them.

Many mathematics educators are surprised why students do not have a correct conception of a winning or a sure-win strategy. I suggest that this is because most of the games that students play in their daily lives have no such strategy, so such an idea contradicts with their real-life experiences.

This finding is in line with what Civil (2002) found out when she played another game called Nim with her students. In this game, there is a sure-win strategy for the player who starts first, but her students mistakenly thought that their ability to win depended on the other players’ moves.

Winning the Game

So, what is the winning strategy for Fifteen?

To solve this game, the students needed to be familiar with the Magic Square and Tic Tac Toe – games they have all played in their childhood.

Magic Square

Magic Square is something that many of our students would have learnt in primary school. In a 3-by-3 Magic Square (see Fig. 1), all three numbers in each row, column and diagonal add up to 15.

Figure 1. Magic Square.

Some of the students did manage to link this game to the Magic Square, but they failed to consider all the possibilities: Are there anymore combinations of three numbers whose sum is 15, other than the eight combinations as shown on the Magic Square?

To win this game, you need to prove that there are no more combinations. Only then can you apply the winning strategy for Tic Tac Toe to the Magic Square. But none of the students were able to link this game to Tic Tac Toe. (In fact, many people do not realize that there is a winning strategy for Tic Tac Toe!)

In the game of Fifteen, a winning strategy for the player who starts first is as follows:

Turn 1: Pick the number 8. If the second player does not pick 5, the first player wins!
Turn 2: If the second player picks 4 the first player can win by picking 6, which will force the second player to pick 1 (to prevent the first player from winning by 8 + 6 + 1 = 15).
Turn 3: The first player can then pick 2 and he will win in two ways: 8 + 2 + 5 = 15 or 6 + 2 + 7 = 15, which the second player cannot prevent.

This looks very confusing but it becomes clearer when you try the above moves as if playing Tic Tac Toe on the Magic Square (see Fig. 2).

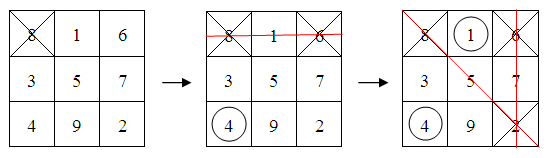
Figure 2. The game of Fifteen.

The challenge, of course, is to play this game without drawing a Magic Square in front of you, to prevent the other player from knowing the winning strategy. This makes the game a lot more complicated and interesting.

Processes for Mathematical Investigation

What are the thinking processes involved when students play mathematically rich games?

To investigate the winning strategy for a game, students have to start by examining specific scenarios or cases (specializing). The next step involves formulating hypotheses or conjectures (conjecturing) and testing them. If the conjectures are proven correct (justifying), they can then be considered as generalizations of the specific cases (generalizing).

These are the four core mathematical thinking processes described by Mason et al. (1985).

For the game Fifteen, if the students are somehow able to see a link between the game and the Magic Square, they would then have some conjecture of how they can win. By confirming it, they can then generalize this to a wider number of cases.

Playing mathematical games such as Fifteen is not just about winning and losing. It is a great way to enrich the learning of math. Plus, it’s a lot of fun!

Resource
Here are some other mathematically rich games that you can use in your math classroom. Download the pdf file.

References
Ainley, J. (1988). Playing games and real mathematics. In D. Pimm (Ed.), Mathematics, teachers and children (pp. 239-248). London: Hodder and Stoughton.

Carraher, D. W., & Schliemann, A. D. (2002). Is everyday mathematics truly relevant to mathematics education? In M. E. Brenner & J. N. Moschkovich (Eds.), Everyday and academic mathematics in the classroom. Journal of Research in Mathematics Education Monograph (No. 11, pp. 131-153). Reston, VA: National Council of Teachers of Mathematics.

Civil, M. (2002). Everyday mathematics, mathematicians’ mathematics, and school mathematics: Can we bring them together? In M. E. Brenner & J. N. Moschkovich (Eds.), Everyday and academic mathematics in the classroom. Journal of Research in Mathematics Education Monograph (No. 11, pp. 40-62). Reston, VA: National Council of Teachers of Mathematics.

Mason, J., Burton, L., & Stacey, K. (1985). Thinking mathematically. Wokingham: Addison-Wesley.
Further readings and resources are available on the SingTeach website.

Further reading
Ainley, J. (1990). Playing games and learning mathematics. In L. P. Steffe & T. Wood (Eds.), Transforming children’s mathematics education: International perspectives (pp. 84-91). Hillsdale, NJ: Erlbaum.

Frobisher, L. (1994). Problems, investigations and an investigative approach. In A. Orton & G. Wain (Eds.), Issues in teaching mathematics (pp. 150-173). London: Cassell.

Pólya, G. (1957). How to solve it: A new aspect of mathematical method (2nd ed.). Princeton, NJ: Princeton University Press.

Skovsmose, O. (2002). Landscapes of investigation. In L. Haggarty (Ed.), Teaching mathematics in secondary schools (pp. 115-128). London: RoutledgeFalmer.